Next: 19.1.7 B97: B97=B97DF +
Up: 19.1 Density Functionals
Previous: 19.1.5 B88:
19.1.6 B95: Becke's 1995 Correlation Functional
A. D. Becke, J. Chem. Phys. 104, 1040 (1995)
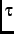 -dependent dynamical correlation functional.
-dependent dynamical correlation functional.
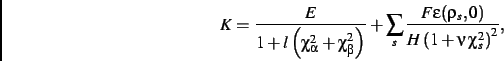 |
(30) |
where
 |
(31) |
 |
(32) |
 |
(33) |
 |
(34) |
 |
(35) |
and
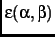 is the correlation energy per particle of the
Local Spin Density Approximation(PW92C).
is the correlation energy per particle of the
Local Spin Density Approximation(PW92C).
molpro@molpro.net
Feb 26, 2003
![]() -dependent dynamical correlation functional.
-dependent dynamical correlation functional.